Regressionandcorrelationcorrelationregressioni

Data: 3.09.2017 / Rating: 4.8 / Views: 922

---

Gallery of Video:

---

Gallery of Images:

---

Regressionandcorrelationcorrelationregressioni

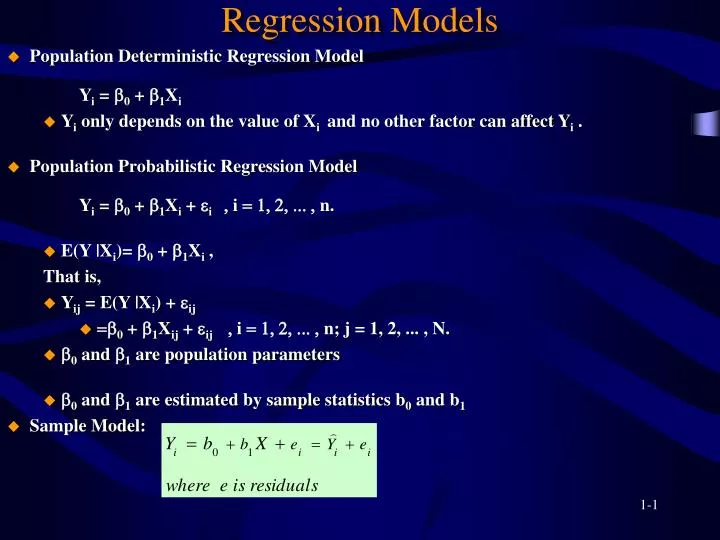
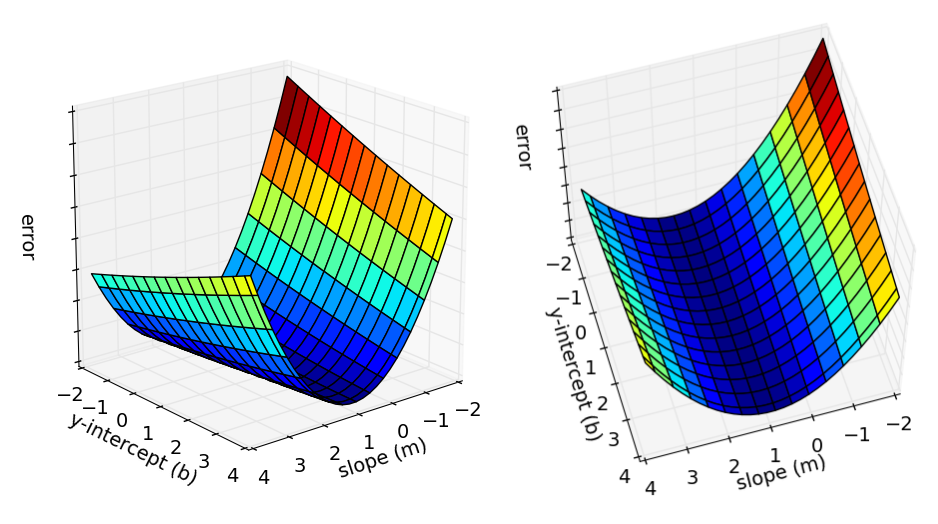
Correlation and linear regression. Use linear regression or correlation when you want to know whether one measurement variable is associated with another measurement variable; you want to measure the strength of the association (r2); or you want an equation that describes the relationship and can be used to predict unknown values. How can the answer be improved. Nov 18, 2012Correlation is a measure of strength of the relationship between two variables. The correlation coefficient quantifies the degree of change in one. 1 NOTES ON CORRELATION AND REGRESSION 1. Correlation Correlation is a measure of association between two variables. The variables are not designated as In statistical modeling, regression analysis is a set of statistical processes for estimating the relationships among variables. It includes many techniques for. Topic 3: Correlation and Regression September 1 and 6, 2011 In this section, we shall take a careful look at the nature of linear relationships found in the data used. Chapter 13Linear Regression and CorrelationTrueFalse 1. If a scatter diagram shows very little scatter about a straight line drawn through the plots. Simple Linear Regression and Correlation 12. 1 The Simple Linear Regression Model 12. 2 Fitting the Regression Line 12. 3 Inferences on the Slope Rarameter. Chapter 10: Regression and Correlation 344 variables are represented as x and y, those labels will be used here. It helps to state which variable is x and which is y. Three main reasons for correlation and regression together are, 1) Test a hypothesis for causality, 2) See association between variables, 3) Estimating a value of a. Introduction to Correlation and Regression Analysis. In this section we will first discuss correlation analysis, which is used to quantify the association between two continuous variables (e. , between an independent and a dependent variable or between two independent variables). Correlation and linear regression are not the same. Correlation quantifies the degree to which two variables are related. Correlation does not Correlation Regression, I 9. 07 Regression and correlation Y, Z, ) Involve bivariate, paired data, X Y Height weight measured for the same Linear Correlation. Linear correlation coefficient is a statistical parameter, r used to define the strength and nature of the linear relationship between Regression and correlation analysis: Regression analysis involves identifying the relationship between a dependent variable and one or more independent variables. A model of the relationship is hypothesized, and estimates of the parameter values are used to develop an estimated regression equation. Correlation and regression analysis are related in the sense that both deal with relationships among variables. The correlation coefficient is a measure of linear association between two variables. Values of the correlation coefficient are always between 1 and 1. Simple Linear Regression and Correlation Menu location: AnalysisRegression and CorrelationSimple Linear and Correlation. I would have expected the correlation coefficient to be the same as a regression slope (beta), however having just compared the two, they are different. Correlation is a measure of association between two variables. The variables are not designated as dependent or independent. The two most popular correlation coefficients are: Spearman's correlation coefficient rho and Pearson's productmoment correlation coefficient. Correlation Regression Chapter 5 Correlation: Do you have a relationship? Between two Quantitative Variables (measured on Same Person) (1) If you have a. Regression and Correlation Menu location: AnalysisRegression and Correlation. Simple linear and correlation; Multiple (general) linear Calculator with step by step explanations to find equation of the regression line and corelation coefficient.

---

Related Images:

---